Toán 10 Bài 8: Tổng và hiệu của hai vectơ Giải SGK Toán 10 trang 54 - Tập 1 sách Kết nối tri thức với cuộc sống

Bài trước

Mục lục

Bài sau

Toán 10 Bài 8 Kết nối tri thức trang 54 giúp các bạn học sinh có thêm nhiều gợi ý tham khảo để trả lời các câu hỏi phần luyện tập và 4 bài tập trong SGK bài Tổng và hiệu của hai vectơ thuộc chương 4 Vectơ.

Giải Toán 10 Kết nối tri thức bài 8 trang 54 được biên soạn với các lời giải chi tiết, đầy đủ và chính xác bám sát chương trình sách giáo khoa môn Toán 10 tập 1. Giải Toán 10 bài 8 Kết nối tri thức là tài liệu cực kì hữu ích hỗ trợ các em học sinh lớp 10 trong quá trình giải bài tập. Đồng thời phụ huynh có thể sử dụng để hướng dẫn con em học tập và đổi mới phương pháp giải phù hợp hơn. Vậy sau đây là nội dung chi tiết giải Toán 10 Bài 8 Tổng và hiệu của hai vectơ mời các bạn cùng theo dõi.

Toán 10 Bài 8: Tổng và hiệu của hai vectơ

Luyện tập Toán 10 Bài 8 Kết nối tri thức

Luyện tập 1

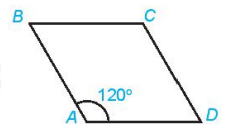
Cho hình thoi ABCD với cạnh có độ dài bằng 1 và $\widehat {BAD} = {120^0}$ $\widehat {BAD} = {120^0}$ . Tính độ dài của các vecto $\overrightarrow {CB} + \overrightarrow {CD} ;\overrightarrow {DB} + \overrightarrow {CD} + \overrightarrow {BA}$ $\overrightarrow {CB} + \overrightarrow {CD} ;\overrightarrow {DB} + \overrightarrow {CD} + \overrightarrow {BA}$

Gợi ý đáp án

Hình vẽ minh họa:

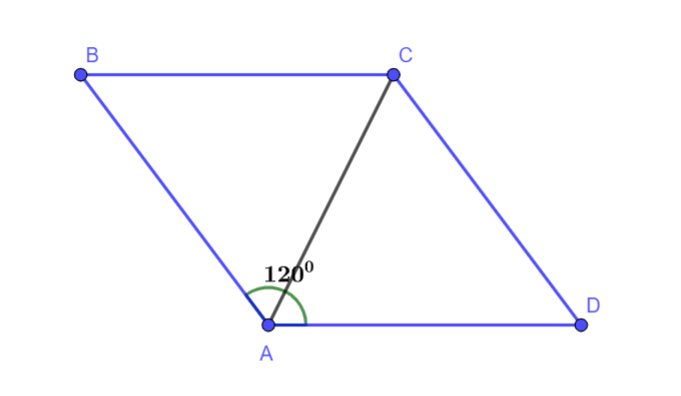
Vì ABCD là hình thoi nên AC là tia phân giác $\widehat {BAC}$ $\widehat {BAC}$

=> $\widehat {BAC} = \widehat {CAD} = \frac{{\widehat {BAD}}}{2} = \frac{{{{120}^0}}}{2} = {60^0}$ $\widehat {BAC} = \widehat {CAD} = \frac{{\widehat {BAD}}}{2} = \frac{{{{120}^0}}}{2} = {60^0}$

Xét tam giác ABC có AB = BC và $\widehat {BAC} = {60^0}$ $\widehat {BAC} = {60^0}$

=> Tam giác ABC đều

=> AC = AB = BC = 1

Ta có:

$\overrightarrow {CB} + \overrightarrow {CD} = \overrightarrow {CA}$ $\overrightarrow {CB} + \overrightarrow {CD} = \overrightarrow {CA}$(Quy tắc hình bình hành)

=> $\left| {\overrightarrow {CB} + \overrightarrow {CD} } \right| = \left| {\overrightarrow {CA} } \right| = CA = 1$ $\left| {\overrightarrow {CB} + \overrightarrow {CD} } \right| = \left| {\overrightarrow {CA} } \right| = CA = 1$

$\overrightarrow {DB} + \overrightarrow {CD} + \overrightarrow {BA} = \overrightarrow {CD} + \overrightarrow {DB} + \overrightarrow {BA}$ $\overrightarrow {DB} + \overrightarrow {CD} + \overrightarrow {BA} = \overrightarrow {CD} + \overrightarrow {DB} + \overrightarrow {BA}$

$= \overrightarrow {CB} + \overrightarrow {BA} = \overrightarrow {CA}$ $= \overrightarrow {CB} + \overrightarrow {BA} = \overrightarrow {CA}$(Quy tắc ba điểm)

=> $\left| {\overrightarrow {DB} + \overrightarrow {CD} + \overrightarrow {BA} } \right| = \left| {\overrightarrow {CA} } \right| = CA = 1$ $\left| {\overrightarrow {DB} + \overrightarrow {CD} + \overrightarrow {BA} } \right| = \left| {\overrightarrow {CA} } \right| = CA = 1$

Luyện tập 2

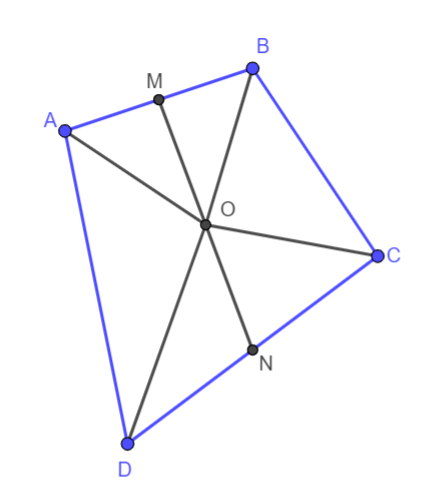
Cho tứ giác ABCD. Gọi M, N lần lượt là trung điểm của các cạnh AB, CD và O là trung điểm của MN. Chứng minh rằng $\overrightarrow {OA} + \overrightarrow {OB} + \overrightarrow {OC} + \overrightarrow {OD} = \overrightarrow 0$ $\overrightarrow {OA} + \overrightarrow {OB} + \overrightarrow {OC} + \overrightarrow {OD} = \overrightarrow 0$

Gợi ý đáp án

Hình vẽ minh họa

Ta có:

$\overrightarrow {OA} + \overrightarrow {OB} = \left( {\overrightarrow {OM} + \overrightarrow {MA} } \right) + \left( {\overrightarrow {OM} + \overrightarrow {MB} } \right)$ $\overrightarrow {OA} + \overrightarrow {OB} = \left( {\overrightarrow {OM} + \overrightarrow {MA} } \right) + \left( {\overrightarrow {OM} + \overrightarrow {MB} } \right)$

=> $\overrightarrow {OA} + \overrightarrow {OB} = \overrightarrow {OM} + \overrightarrow {MA} + \overrightarrow {OM} + \overrightarrow {MB}$ $\overrightarrow {OA} + \overrightarrow {OB} = \overrightarrow {OM} + \overrightarrow {MA} + \overrightarrow {OM} + \overrightarrow {MB}$

=> $\overrightarrow {OA} + \overrightarrow {OB} = 2\overrightarrow {OM} + \left( {\overrightarrow {MA} + \overrightarrow {MB} } \right)$ $\overrightarrow {OA} + \overrightarrow {OB} = 2\overrightarrow {OM} + \left( {\overrightarrow {MA} + \overrightarrow {MB} } \right)$

Do M là trung điểm của AB

=> $\overrightarrow {OA} + \overrightarrow {OB} = 2\overrightarrow {OM} + \overrightarrow 0 = 2\overrightarrow {OM}$ $\overrightarrow {OA} + \overrightarrow {OB} = 2\overrightarrow {OM} + \overrightarrow 0 = 2\overrightarrow {OM}$

$\overrightarrow {OC} + \overrightarrow {OD} = \left( {\overrightarrow {ON} + \overrightarrow {NC} } \right) + \left( {\overrightarrow {ON} + \overrightarrow {ND} } \right)$ $\overrightarrow {OC} + \overrightarrow {OD} = \left( {\overrightarrow {ON} + \overrightarrow {NC} } \right) + \left( {\overrightarrow {ON} + \overrightarrow {ND} } \right)$

=> $\overrightarrow {OC} + \overrightarrow {OD} = \overrightarrow {ON} + \overrightarrow {NC} + \overrightarrow {ON} + \overrightarrow {ND}$ $\overrightarrow {OC} + \overrightarrow {OD} = \overrightarrow {ON} + \overrightarrow {NC} + \overrightarrow {ON} + \overrightarrow {ND}$

=> $\overrightarrow {OC} + \overrightarrow {OD} = 2\overrightarrow {ON} + \overrightarrow {NC} + \overrightarrow {ND}$ $\overrightarrow {OC} + \overrightarrow {OD} = 2\overrightarrow {ON} + \overrightarrow {NC} + \overrightarrow {ND}$

=> $\overrightarrow {OC} + \overrightarrow {OD} = 2\overrightarrow {ON} + \left( {\overrightarrow {NC} + \overrightarrow {ND} } \right)$ $\overrightarrow {OC} + \overrightarrow {OD} = 2\overrightarrow {ON} + \left( {\overrightarrow {NC} + \overrightarrow {ND} } \right)$

Do N là trung điểm của CD

=> $\overrightarrow {OC} + \overrightarrow {OD} = 2\overrightarrow {ON} + \overrightarrow 0 = 2\overrightarrow {ON}$ $\overrightarrow {OC} + \overrightarrow {OD} = 2\overrightarrow {ON} + \overrightarrow 0 = 2\overrightarrow {ON}$

Khi đó suy ra: $\overrightarrow {OA} + \overrightarrow {OB} + \overrightarrow {OC} + \overrightarrow {OD} = 2\overrightarrow {OM} + 2\overrightarrow {ON}$ $\overrightarrow {OA} + \overrightarrow {OB} + \overrightarrow {OC} + \overrightarrow {OD} = 2\overrightarrow {OM} + 2\overrightarrow {ON}$

Do O là trung điểm của MN

=> $2\overrightarrow {OM} + 2\overrightarrow {ON} = \overrightarrow 0$ $2\overrightarrow {OM} + 2\overrightarrow {ON} = \overrightarrow 0$

=> $\overrightarrow {OA} + \overrightarrow {OB} + \overrightarrow {OC} + \overrightarrow {OD} = \overrightarrow 0$ $\overrightarrow {OA} + \overrightarrow {OB} + \overrightarrow {OC} + \overrightarrow {OD} = \overrightarrow 0$

=> Điều phải chứng minh

Giải Toán 10 trang 54 Kết nối tri thức tập 1

Bài 4.6 trang 54

Cho bốn điểm A, B, C, D. Chứng minh rằng:

a) $\overrightarrow {AB} + \overrightarrow {BC} + \overrightarrow {CD} + \overrightarrow {DA} = \overrightarrow 0$ $\overrightarrow {AB} + \overrightarrow {BC} + \overrightarrow {CD} + \overrightarrow {DA} = \overrightarrow 0$

$b) \overrightarrow {AC} - \overrightarrow {AD} = \overrightarrow {BC} - \overrightarrow {BD}$ $b) \overrightarrow {AC} - \overrightarrow {AD} = \overrightarrow {BC} - \overrightarrow {BD}$

Gợi ý đáp án

$\begin{array}{l}\overrightarrow {AB} + \overrightarrow {BC} + \overrightarrow {CD} + \overrightarrow {DA} = \left( {\overrightarrow {AB} + \overrightarrow {BC} } \right) + \left( {\overrightarrow {CD} + \overrightarrow {DA} } \right)\\ = \overrightarrow {AC} + \overrightarrow {CA} = \overrightarrow {AA} = \overrightarrow 0 .\end{array}$ $\begin{array}{l}\overrightarrow {AB} + \overrightarrow {BC} + \overrightarrow {CD} + \overrightarrow {DA} = \left( {\overrightarrow {AB} + \overrightarrow {BC} } \right) + \left( {\overrightarrow {CD} + \overrightarrow {DA} } \right)\\ = \overrightarrow {AC} + \overrightarrow {CA} = \overrightarrow {AA} = \overrightarrow 0 .\end{array}$

$\overrightarrow {AC} - \overrightarrow {AD} = \overrightarrow {DC} và \overrightarrow {BC} - \overrightarrow {BD} = \overrightarrow {DC} \Rightarrow \overrightarrow {AC} - \overrightarrow {AD} = \overrightarrow {BC} - \overrightarrow {BD}$ $\overrightarrow {AC} - \overrightarrow {AD} = \overrightarrow {DC} và \overrightarrow {BC} - \overrightarrow {BD} = \overrightarrow {DC} \Rightarrow \overrightarrow {AC} - \overrightarrow {AD} = \overrightarrow {BC} - \overrightarrow {BD}$

Bài 4.7 trang 54

Cho hình bình hành ABCD. Hãy tìm điểm M để $\overrightarrow {BM} = \overrightarrow {AB} + \overrightarrow {AD}$ $\overrightarrow {BM} = \overrightarrow {AB} + \overrightarrow {AD}$. Tìm mối quan hệ giữa hai vectơ $\overrightarrow {CD} và \overrightarrow {CM} .$ $\overrightarrow {CD} và \overrightarrow {CM} .$

Gợi ý đáp án

Ta có: $\overrightarrow {AD} = \overrightarrow {BC}$ $\overrightarrow {AD} = \overrightarrow {BC}$(do ABCD là hình bình hành)

$\Rightarrow \overrightarrow {AB} + \overrightarrow {AD} = \overrightarrow {AB} + \overrightarrow {BC} = \overrightarrow {AC}$ $\Rightarrow \overrightarrow {AB} + \overrightarrow {AD} = \overrightarrow {AB} + \overrightarrow {BC} = \overrightarrow {AC}$

$\Rightarrow \overrightarrow {BM} = \overrightarrow {AB} + \overrightarrow {AD} = \overrightarrow {AC}$ $\Rightarrow \overrightarrow {BM} = \overrightarrow {AB} + \overrightarrow {AD} = \overrightarrow {AC}$

$\Rightarrow$ $\Rightarrow$Tứ giác ABMC là hình bình hành.

$\Rightarrow \overrightarrow {AB} = \overrightarrow {CM} . Mà \overrightarrow {AB} = \overrightarrow {DC}$ $\Rightarrow \overrightarrow {AB} = \overrightarrow {CM} . Mà \overrightarrow {AB} = \overrightarrow {DC}$

$\Rightarrow \overrightarrow {DC} = \overrightarrow {CM}$ $\Rightarrow \overrightarrow {DC} = \overrightarrow {CM}$

$\Rightarrow C$ $\Rightarrow C$ là trung điểm DM.

Nói cách khác: $\overrightarrow {CD} + \overrightarrow {CM} = \overrightarrow 0$ $\overrightarrow {CD} + \overrightarrow {CM} = \overrightarrow 0$hay hai vectơ $\overrightarrow {CD} và \overrightarrow {CM}$ $\overrightarrow {CD} và \overrightarrow {CM}$ đối nhau.

Bài 4.8 trang 54

Cho tam giác đều ABC có cạnh bằng a. Tính độ dài của các vectơ $\overrightarrow {AB} - \overrightarrow {AC} ,\;\overrightarrow {AB} + \overrightarrow {AC} .$ $\overrightarrow {AB} - \overrightarrow {AC} ,\;\overrightarrow {AB} + \overrightarrow {AC} .$

Gợi ý đáp án

$\overrightarrow {AB} - \overrightarrow {AC} = \overrightarrow {CB} \Rightarrow \left| {\overrightarrow {AB} - \overrightarrow {AC} } \right| = \left| {\overrightarrow {CB} } \right| = CB = a.$ $\overrightarrow {AB} - \overrightarrow {AC} = \overrightarrow {CB} \Rightarrow \left| {\overrightarrow {AB} - \overrightarrow {AC} } \right| = \left| {\overrightarrow {CB} } \right| = CB = a.$

Dựng hình bình hành ABDC tâm O như hình vẽ.

Ta có:

$\overrightarrow {AB} + \overrightarrow {AC} = \overrightarrow {AB} + \overrightarrow {BD} = \overrightarrow {AD}$ $\overrightarrow {AB} + \overrightarrow {AC} = \overrightarrow {AB} + \overrightarrow {BD} = \overrightarrow {AD}$

$\Rightarrow \left| {\overrightarrow {AB} + \overrightarrow {AC} } \right| = \left| {\overrightarrow {AD} } \right| = AD$ $\Rightarrow \left| {\overrightarrow {AB} + \overrightarrow {AC} } \right| = \left| {\overrightarrow {AD} } \right| = AD$

Vì tứ giác ABDC là hình bình hành, lại có AB = AC = BD = CD = a nên ABDC là hình thoi.

$\Rightarrow AD = 2AO = 2.AB.\sin B = 2a.\frac{{\sqrt 3 }}{2} = a\sqrt 3 .$ $\Rightarrow AD = 2AO = 2.AB.\sin B = 2a.\frac{{\sqrt 3 }}{2} = a\sqrt 3 .$

Vậy $\left| {\overrightarrow {AB} - \overrightarrow {AC} } \right| = a và \left| {\overrightarrow {AB} + \overrightarrow {AC} } \right| = a\sqrt 3 .$ $\left| {\overrightarrow {AB} - \overrightarrow {AC} } \right| = a và \left| {\overrightarrow {AB} + \overrightarrow {AC} } \right| = a\sqrt 3 .$

Bài 4.9 trang 54

Hình 4.19 biểu diễn hai lực $\overrightarrow {{F_1}} ,\overrightarrow {{F_2}}$ $\overrightarrow {{F_1}} ,\overrightarrow {{F_2}}$cùng tác động lên một vật, cho $\left| {\overrightarrow {{F_1}} } \right| = 3\;N,\;\left| {\overrightarrow {{F_2}} } \right| = 2\;N$ $\left| {\overrightarrow {{F_1}} } \right| = 3\;N,\;\left| {\overrightarrow {{F_2}} } \right| = 2\;N$. Tính độ lớn của hợp lực $\overrightarrow {{F_1}} + \overrightarrow {{F_2}} .$ $\overrightarrow {{F_1}} + \overrightarrow {{F_2}} .$

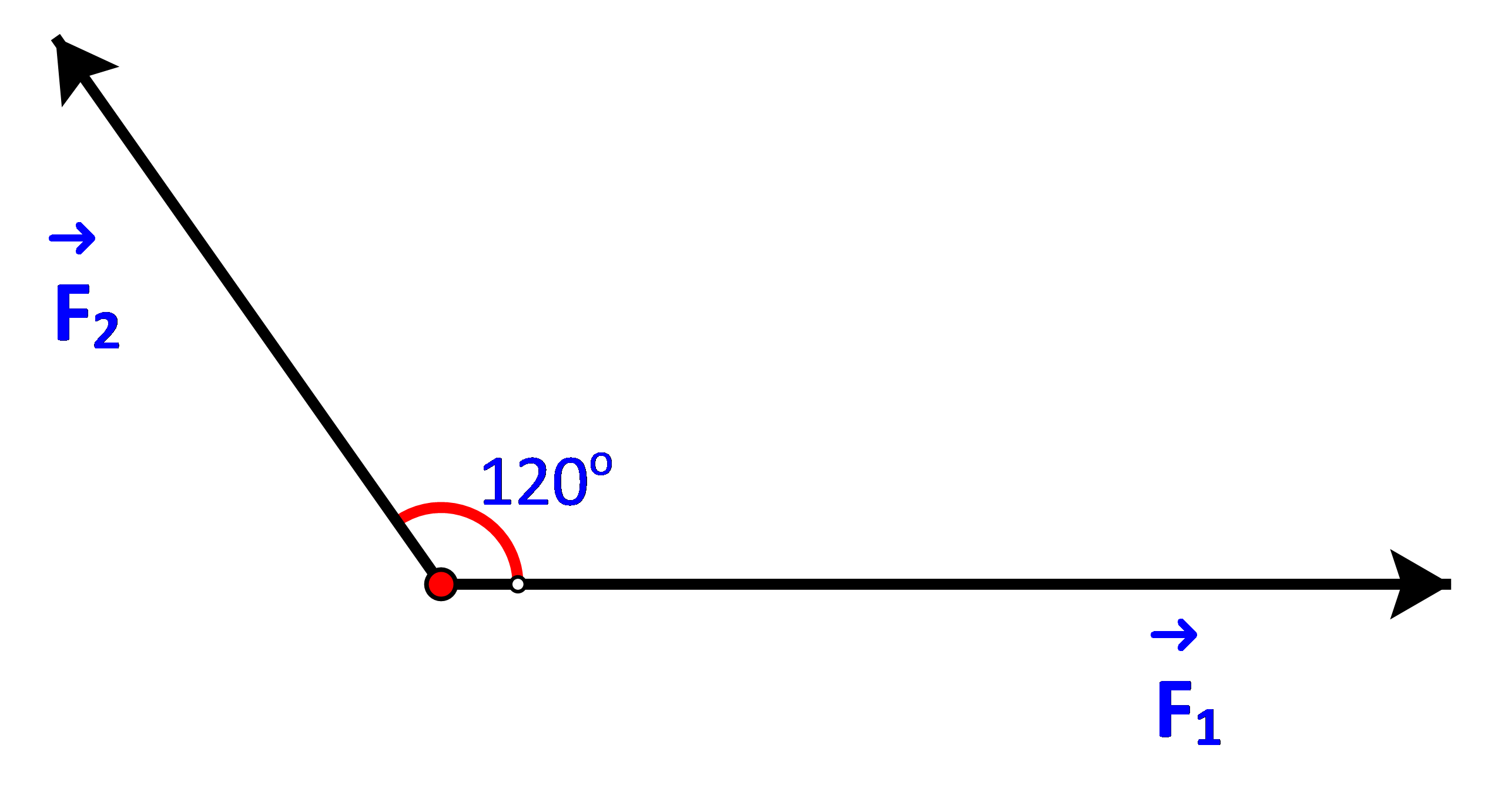
Gợi ý đáp án

Dựng hình bình hành ABDC với hai cạnh là hai vectơ $\overrightarrow {{F_1}} ,\overrightarrow {{F_2}}$ $\overrightarrow {{F_1}} ,\overrightarrow {{F_2}}$như hình vẽ

Ta có:

$\overrightarrow {{F_1}} + \overrightarrow {{F_2}} = \overrightarrow {AC} + \overrightarrow {AB} = \overrightarrow {AD} \Rightarrow \left| {\overrightarrow {{F_1}} + \overrightarrow {{F_2}} } \right| = \left| {\overrightarrow {AD} } \right| = AD$ $\overrightarrow {{F_1}} + \overrightarrow {{F_2}} = \overrightarrow {AC} + \overrightarrow {AB} = \overrightarrow {AD} \Rightarrow \left| {\overrightarrow {{F_1}} + \overrightarrow {{F_2}} } \right| = \left| {\overrightarrow {AD} } \right| = AD$

Xét $\Delta$ $\Delta$ ABD ta có:

$BD = AC = \left| {\overrightarrow {{F_1}} } \right| = 3\;,AB = \;\left| {\overrightarrow {{F_2}} } \right| = 2\;.$ $BD = AC = \left| {\overrightarrow {{F_1}} } \right| = 3\;,AB = \;\left| {\overrightarrow {{F_2}} } \right| = 2\;.$

$\widehat {ABD} = {180^o} - \widehat {BAC} = {180^o} - {120^o} = {60^o}$ $\widehat {ABD} = {180^o} - \widehat {BAC} = {180^o} - {120^o} = {60^o}$

Theo định lí cosin ta có:

$\begin{array}{l}A{D^2} = A{B^2} + B{D^2} - 2.AB.BD.\cos \widehat {ABD}\\ \Leftrightarrow A{D^2} = {2^2} + {3^2} - 2.2.3.\cos {120^o}\\ \Leftrightarrow A{D^2} = 19\\ \Leftrightarrow AD = \sqrt {19} \end{array}$ $\begin{array}{l}A{D^2} = A{B^2} + B{D^2} - 2.AB.BD.\cos \widehat {ABD}\\ \Leftrightarrow A{D^2} = {2^2} + {3^2} - 2.2.3.\cos {120^o}\\ \Leftrightarrow A{D^2} = 19\\ \Leftrightarrow AD = \sqrt {19} \end{array}$

Vậy $\left| {\overrightarrow {{F_1}} + \overrightarrow {{F_2}} } \right| = \sqrt {19}$ $\left| {\overrightarrow {{F_1}} + \overrightarrow {{F_2}} } \right| = \sqrt {19}$

Lý thuyết Tổng và hiệu của hai vectơ

1. Tổng của hai vectơ

Định nghĩa: Cho hai vectơ $\overrightarrow{a}, \overrightarrow{b}$ $\overrightarrow{a}, \overrightarrow{b}$. Lấy một điểm A tùy ý, vẽ $\overrightarrow{AB} = \overrightarrow{a}, \overrightarrow{BC} = \overrightarrow{b}$ $\overrightarrow{AB} = \overrightarrow{a}, \overrightarrow{BC} = \overrightarrow{b}$. Vectơ $\overrightarrow{AC}$ $\overrightarrow{AC}$ được gọi là tổng của hai vectơ $\overrightarrow{a}$ $\overrightarrow{a}$ và $\overrightarrow{b}.$ $\overrightarrow{b}.$

$\overrightarrow{AC} = \overrightarrow{a} + \overrightarrow{b}.$ $\overrightarrow{AC} = \overrightarrow{a} + \overrightarrow{b}.$

2. Quy tắc hình bình hành

Nếu ABCD là hình bình hành thì

$\overrightarrow{AB} + \overrightarrow{AD} = \overrightarrow{AC}.$ $\overrightarrow{AB} + \overrightarrow{AD} = \overrightarrow{AC}.$

3. Tính chất của tổng các vectơ

- Tính chất giao hoán

$\overrightarrow{a} + \overrightarrow{b} = \overrightarrow{b} + \overrightarrow{a}$ $\overrightarrow{a} + \overrightarrow{b} = \overrightarrow{b} + \overrightarrow{a}$

- Tính chất kết hợp

$(\overrightarrow{a} + \overrightarrow{b} ) + \overrightarrow{c} = \overrightarrow{a} + (\overrightarrow{b} +\overrightarrow{c})$ $(\overrightarrow{a} + \overrightarrow{b} ) + \overrightarrow{c} = \overrightarrow{a} + (\overrightarrow{b} +\overrightarrow{c})$

- Tính chất của $\overrightarrow{0}$ $\overrightarrow{0}$:

$\overrightarrow{a}+\overrightarrow{0} = \overrightarrow{0} + \overrightarrow{a} =\overrightarrow{a}$ $\overrightarrow{a}+\overrightarrow{0} = \overrightarrow{0} + \overrightarrow{a} =\overrightarrow{a}$

II. Hiệu của hai vectơ

a) Vec tơ đối: Vectơ có cùng độ dài và ngược hướng với vec tơ $\overrightarrow{a}$ $\overrightarrow{a}$ được gọi là vec tơ đối của vec tơ $\overrightarrow{a}$ $\overrightarrow{a}$ , kí hiệu $-\overrightarrow{a}.$ $-\overrightarrow{a}.$

Vec tơ đối của $\overrightarrow{0}$ $\overrightarrow{0}$ là vectơ $\overrightarrow{0}.$ $\overrightarrow{0}.$

b) Hiệu của hai vec tơ: Cho hai vectơ $\overrightarrow{a}, \overrightarrow{b}$ $\overrightarrow{a}, \overrightarrow{b}$. Vec tơ hiệu của hai vectơ, kí hiệu $\overrightarrow{a}- \overrightarrow{b}$ $\overrightarrow{a}- \overrightarrow{b}$ là vectơ $\overrightarrow{a} + (-\overrightarrow{b})$ $\overrightarrow{a} + (-\overrightarrow{b})$

$\overrightarrow{a}- \overrightarrow{b} = \overrightarrow{a} + (-\overrightarrow{b}).$ $\overrightarrow{a}- \overrightarrow{b} = \overrightarrow{a} + (-\overrightarrow{b}).$

c) Chú ý: Với ba điểm bất kì, ta luôn có

$\overrightarrow{AB} + \overrightarrow{BC} = \overrightarrow{AC} (1)$ $\overrightarrow{AB} + \overrightarrow{BC} = \overrightarrow{AC} (1)$

$\overrightarrow{AB} - \overrightarrow{AC} = \overrightarrow{CB} (2)$ $\overrightarrow{AB} - \overrightarrow{AC} = \overrightarrow{CB} (2)$

(1) là quy tắc 3 điểm (quy tắc tam giác) đối với tổng của hai vectơ.

(2) là quy tắc 3 điểm (quy tắc tam giác) đối với hiệu các vectơ.

Chia sẻ bởi: